We come across various equations when studying mathematics. In arithmetic, there are many different types of equations, including linear and quadratic equations. Equations are taught in elementary school and are utilized in advanced mathematics as well. Equations have a wide range of real-world applications; we utilize them to locate variables in various domains. A quadratic equation is one of the most commonly utilized equations. Let’s talk about quadratic equations, their characteristics, and study in detail the quadratic formula used to solve the equation.

Quadratic Equation:

Quadratic equations are one of the subsets of equations. There are many different types of equations that we deal with in mathematics. A quadratic equation is the one that has the highest power of the variable as two. Let us take an example of a quadratic equation, consider the equation ax2+bx+c=0. Here a, b, and all are constant. In the equation mentioned, the variable of x2 that is ‘a’ should never be equal to zero. It is a necessary condition for a quadratic equation. Also, we can notice in the equation that the highest power of the variable is two. There are different methods to solve a quadratic equation. Let us discuss one of the formulas used to find the solution of a quadratic equation. We have to find the answers to the equation, by finding the values of variables. The values of the variables that are the solution to the equation are also called the roots of the equation.

Solving quadratic equations:

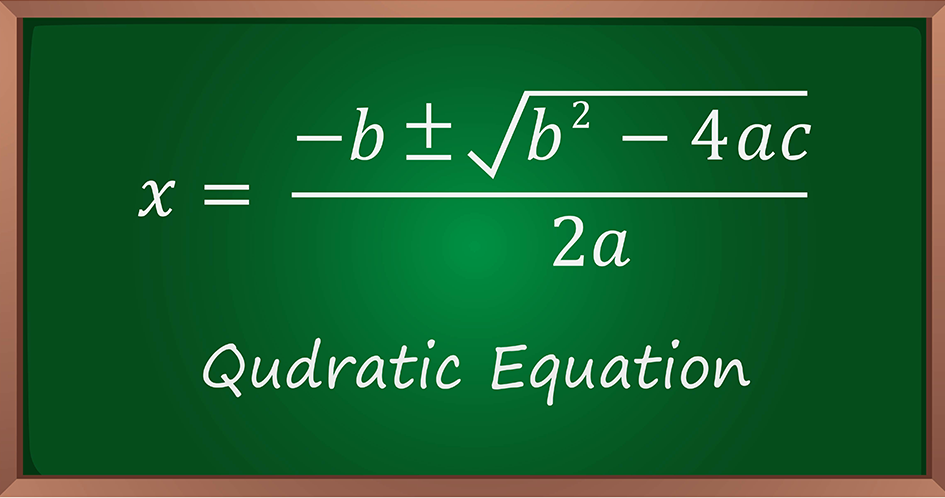
Quadratic formula is one of the easiest methods to find the solution of a quadratic equation. Let us take the above-mentioned equation for further explanation. Let the roots of the above-mentioned equation be alpha and beta. [-b ± √ (b² – 4ac)]/2a is the quadratic formula used to find the roots. Using this formula, we will be getting two roots alpha and beta of the quadratic equation. Both the roots that we get from the formula will satisfy the equation. b2 – 4ac used in the quadratic formula is called the discriminant. The discriminant is of huge importance in the formula and gives us the idea of the type of roots. If the discriminant of the equation is equal to zero, then both the roots of the equation will be real and equal in value. Similarly, if the discriminant is greater than zero, then both the roots will be real and different from each other. At last, if the discriminant is smaller than zero, then the roots for the equation are not equal and are imaginary. A student should learn this formula by heart as it is very crucial in solving quadratic equations. Let us discuss a few of the properties shown by these equations.

Graph of every equation holds great importance in mathematics. Graph of a quadratic equation, when plotted, is always parabola. The type of parabola that will be formed depends on the equation. If the equation is quadratic in x, then the parabola will be different from the parabola that will be formed if the equation is quadratic in y. One more property of the equation is, that the sum of the two roots that is alpha and beta is always equal to -b/a where a is the coefficient of x2 and b is the value of the coefficient of x. Similarly, the product of the roots is c/a, where c is the constant and a is the coefficient of x2. All these properties should be understood by students in-depth as they are of great use.

In the above article, we have discussed quadratic equations and quadratic formulas, a method of solving quadratic equations. If any student faces a problem in solving such a maths-related topic, then they should take the help of an online platform such as Cuemath. Cuemath provides students with one of the best education in math and coding.

solving quadratic equations

admin